HDU 4347 The Closest M Points （KD树）

HDU4347

KD树模板题，求K近邻，暴力，加个优先队列。

The Closest M Points

Time Limit: 16000/8000 MS (Java/Others) Memory Limit: 98304/98304 K (Java/Others) Total Submission(s): 1963 Accepted Submission(s): 786

Problem Description

The course of Software Design and Development Practice is objectionable. ZLC is facing a serious problem .There are many points in K-dimensional space .Given a point. ZLC need to find out the closest m points. Euclidean distance is used as the distance metric between two points. The Euclidean distance between points p and q is the length of the line segment connecting them.In Cartesian coordinates, if p = (p1, p2,…, pn) and q = (q1, q2,…, qn) are two points in Euclidean n-space, then the distance from p to q, or from q to p is given by:

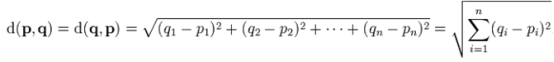
Can you help him solve this problem?

Input

In the first line of the text file .there are two non-negative integers n and K. They denote respectively: the number of points, 1 <= n <= 50000, and the number of Dimensions,1 <= K <= 5. In each of the following n lines there is written k integers, representing the coordinates of a point. This followed by a line with one positive integer t, representing the number of queries,1 <= t <=10000.each query contains two lines. The k integers in the first line represent the given point. In the second line, there is one integer m, the number of closest points you should find,1 <= m <=10. The absolute value of all the coordinates will not be more than 10000. There are multiple test cases. Process to end of file.

Output

For each query, output m+1 lines: The first line saying :”the closest m points are:” where m is the number of the points. The following m lines representing m points ,in accordance with the order from near to far It is guaranteed that the answer can only be formed in one ways. The distances from the given point to all the nearest m+1 points are different. That means input like this: 2 2 1 1 3 3 1 2 2 1 will not exist.

Sample Input

3 2 1 1 1 3 3 4 2 2 3 2 2 3 1

Sample Output

the closest 2 points are: 1 3 3 4 the closest 1 points are: 1 3

Author

HIT

Source

2012 Multi-University Training Contest 5

/* ***************

Author        :kuangbin

Created Time  :2014/9/6 18:26:05

File Name     :E:\2014ACM\专题学习\KD树\HDU4347.cpp

 ************************************************ */

#include <stdio.h>

#include <string.h>

#include <iostream>

#include <algorithm>

#include <vector>

#include <queue>

#include <set>

#include <map>

#include <string>

#include <math.h>

#include <stdlib.h>

#include <time.h>

using namespace std;

const int MAXN = 50010;

const int DIM = 10;

inline double sqr(double x){return x*x;}

namespace KDTree{

	int K;//维数

	struct Point{

		int x[DIM];

		double distance(const Point &b)const{

			double ret = 0;

			for(int i = 0;i < K;i++)

				ret += sqr(x[i]-b.x[i]);

			return ret;

		}

		void input(){

			for(int i = 0;i < K;i++)scanf("%d",&x[i]);

		}

		void output(){

			for(int i = 0;i < K;i++)

				printf("%d%c",x[i],i < K-1?' ':'\n');

		}

	};

	struct qnode{

		Point p;

		double dis;

		qnode(){}

		qnode(Point _p,double _dis){

			p = _p; dis = _dis;

		}

		bool operator <(const qnode &b)const{

			return dis < b.dis;

		}

	};

	priority_queue<qnode>q;

	struct cmpx{

		int div;

		cmpx(const int &_div){div = _div;}

		bool operator()(const Point &a,const Point &b){

			for(int i = 0;i < K;i++)

				if(a.x[(div+i)%K] != b.x[(div+i)%K])

					return a.x[(div+i)%K] < b.x[(div+i)%K];

			return true;

		}

	};

	bool cmp(const Point &a,const Point &b,int div){

		cmpx cp = cmpx(div);

		return cp(a,b);

	}

	struct Node{

		Point e;

		Node *lc,*rc;

		int div;

	}pool[MAXN],*tail,*root;

	void init(){

		tail = pool;

	}

	Node* build(Point *a,int l,int r,int div){

		if(l >= r)return NULL;

		Node *p = tail++;

		p->div = div;

		int mid = (l+r)/2;

		nth_element(a+l,a+mid,a+r,cmpx(div));

		p->e = a[mid];

		p->lc = build(a,l,mid,(div+1)%K);

		p->rc = build(a,mid+1,r,(div+1)%K);

		return p;

	}

	void search(Point p,Node *x,int div,int m){

		if(!x)return;

		if(cmp(p,x->e,div)){

			search(p,x->lc,(div+1)%K,m);

			if(q.size() < m){

				q.push(qnode(x->e,p.distance(x->e)));

				search(p,x->rc,(div+1)%K,m);

			}

			else {

				if(p.distance(x->e) < q.top().dis){

					q.pop(); 

					q.push(qnode(x->e,p.distance(x->e)));

				}

				if(sqr(x->e.x[div]-p.x[div]) < q.top().dis)

					search(p,x->rc,(div+1)%K,m);

			}

		}

		else {

			search(p,x->rc,(div+1)%K,m);

			if(q.size() < m){

				q.push(qnode(x->e,p.distance(x->e)));

				search(p,x->lc,(div+1)%K,m);

			}

			else {

				if(p.distance(x->e) < q.top().dis){

					q.pop();

					q.push(qnode(x->e,p.distance(x->e)));

				}

				if(sqr(x->e.x[div]-p.x[div]) < q.top().dis)

					search(p,x->lc,(div+1)%K,m);

			}

		}

	}

	void search(Point p,int m){

		while(!q.empty())q.pop();

		search(p,root,0,m);

	}

};

KDTree::Point p[MAXN];

int main()

{

	int n,k;

	while(scanf("%d%d",&n,&k) == 2){

		KDTree::K = k;

		for(int i = 0;i < n;i++)p[i].input();

		KDTree::init();

		KDTree::root = KDTree::build(p,0,n,0);

		int Q;

		scanf("%d",&Q);

		KDTree::Point o;

		while(Q--){

			o.input();

			int m;

			scanf("%d",&m);

			KDTree::search(o,m);

			printf("the closest %d points are:\n",m);

			int cnt = 0;

			while(!KDTree::q.empty()){

				p[cnt++] = KDTree::q.top().p;

				KDTree::q.pop();

			}

			for(int i = 0;i < m;i++)p[m-1-i].output();

		}

	}

	return 0;

}